A perfect balance

These days, the Government of Botswana's Department of Taxes and Attorney General's Chambers is no doubt the setting for much balancing of figures and weighing up of legal issues. But rewind a few years and balancing valves, not numbers, was the big issue in the low-energy building scheme.

The competition-winning bid for the 16-storey building (pictured above) in Gaborone, Botswana's capital, came from a design team led by Pramod Patel Architects.

As part of the low energy approach, the team set a cooling load target of 120 W/m², rather than the 180-200 W/m² typically found in other buildings in the region. This is no mean feat in a semi-arid country in Southern Africa, where ambient summer temperatures often exceed 40°C - but three years after the building swung into operation, the installed cooling load of 112 W/m² has bettered the original target. So how did they do it?

The geometry of the multifaceted building meant that extensive 3D shade modelling formed part of the design process. The design of the facade received particular attention, with heat gain limited by the use of solar control double-glazed units (containing a low emissivity inner pane) and heavily insulated non-vision panels. Roof and floor overhangs provided additional shading.

The M&E team had to design an air conditioning system that would complement the thermal efficiency of this facade and respond efficiently to the building's variations in load. The solution we came up with was to use a self-balancing chilled water pipework system with variable speed pumps. The system is used to connect a rooftop chiller plant to sixty-two variable air volume and four constant volume air-handling units located throughout the building.

The variable speed design method was first proposed in the early 1990s and reported in the American ASHRAE journal. This was the first time the engineers in Botswana had used the system.

There are significant benefits to variable speed pumping in terms of both energy cost and capital cost. The main energy savings derive from the pump law relationship which states that, for a system with fixed resistance, halving the flow rate would reduce the pump power to roughly one eighth of its previous value. The reasons for this become obvious when you consider the relationship between pump power, pump head and flow rate. The power consumed by a pump can be calculated as follows:

P = rgHQ

h

where P = pump power (W)

r = density (kg/m3)

g = gravitational constant

H = pump head (m; equivalent to pressure)

Q = flow rate (m3/s)

h = pump efficiency.

By getting rid of all flow-balancing valves and effectively letting the system run unbalanced in its full load condition, the pump pressure can be controlled such that under part load conditions, both pump pressure and flow rate can be allowed to fall. This is because, as system flow rates fall, any initial imbalance in branch pipework pressures tends to even out.

Using this method provides significant capital cost-saving opportunities. The installer avoids the capital cost of flow balancing valves and differential pressure control valves. It also allows three-port valves, which divert flow away from terminal units, to be replaced by two-port valves to modulate branch flows down to zero.

Careful calculations

Constant flow chilled water systems allow engineers to predict full flow design conditions so that they can select components such as pumps, valves and terminal units accordingly. However, in

systems where there is the facility to vary pump speed, many of these certainties are removed and the engineer is forced to take into consideration part load operating conditions.

Our challenge was to predict the likely operating conditions in the system under full and part load, to ensure they avoided hydraulic problems such as noise or cavitation while maintaining good control valve authorities (the ratio between pressure drop across the valve when fully open compared to when it is fully closed). Also, too little flow to some terminals could have caused prolonged cooling periods at start-up, while high flow rates could result in excessive velocities and noise.

To allow the system to be operated in an unbalanced condition required some initial calculation of the natural balance of flows that would occur around it. This way, we could size the pumps and ensure that the degree of under-flows and over-flows were within acceptable limits. This calculation, carried out using network analysis software developed by Chris Parsloe and issued by BSRIA in the late 1990s, enabled the designers to identify potential problems and modify the design accordingly.

Self-balancing act

To encourage a greater degree of self-balancing, mains pipe sizes were increased where necessary. Also, the terminal unit coils were deliberately selected to limit the difference in pressure drops between them to less than 15–20 kPa.

Two-port control valves were also selected to improve the self-balancing properties of the system. Overall branch pressure losses were equalised by selecting two-port valves so that combined terminal unit and two-port valve pressure losses were roughly the same for each terminal branch. To achieve this, we specified valves with a wide range of flow coefficient (kv) values.

For each two-port valve, the highest possible valve authority was achieved by sizing against the pressure losses in the coil and adjoining pipes. By leaving out the flow balancing valves, it was easier to select two port valves with good authority because they no longer had to compete for authority with the balancing valves.

We also checked two-port valve selections to ensure that the maximum velocities through them under full and part load conditions were low enough to avoid possible noise or conditions where cavitation might occur – although this risk had been largely eliminated because the pump pressure would fall under part load conditions, in any case. For this reason, there was no need for differential pressure control valves to protect the two-port valves.

As a rule of thumb, to avoid cavitation the maximum overall pressure drop across a two-port control valve must comply with the following rule:

DP < Km (P1 – Pc)

where DP = overall pressure drop across the valve

P1 = absolute pressure at inlet to the valve

Pc = vapour pressure of the liquid

Km = valve recovery coefficient.

If cavitation was likely to happen, it would probably have occurred on the upper floors, where the system's absolute pressure would be lowest and the pump pressure highest. Increasing the system's static pressure through the pressurisation unit can usually solve problems of this kind.

Further flow regulation

The terminal unit coils were selected to ensure laminar flow would not occur until flow rates had dropped below 30% of their design values. This enabled a fall to between 45–50% of the coils' full load outputs before the turbulent flow entered a transitional phase and the coil output became less predictable.

The scheme had two chilled water circuits. A primary circuit was installed with constant speed pumps to maintain constant flow through the chillers, while a secondary circuit contained the two variable speed pumps. The secondary's pumps were each sized at +/- 50% design flow and were arranged in parallel. They were selected with the intention of running them both at high load, and only one at low load to give greater savings at part load conditions. By using two pumps, it was possible to reduce the flow to 25% of the design total flow while maintaining good pump efficiency.

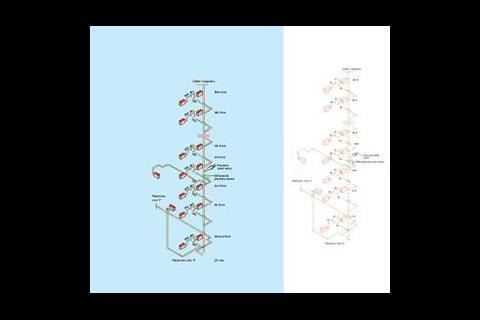
In order to control the secondary circuit's pump speed, a differential pressure sensor was located across the flow and return mains approximately halfway down the system. This was located at a point indicated by the BSRIA software as the mid-point of the system (in terms of pressure loss) – ie the point at which, in its unbalanced state, positive branch flows turned to negative (see diagram, page 67). By controlling the differential pressure constant at this point, excess flows upstream of the sensor would reduce under part load conditions, while low flows downstream would increase.

A bypass with a pressure relief valve was installed at the same mid-point location. The pressure relief valve was set to open at a pressure slightly higher than the constant differential pressure controlled by the pump. This meant that when the pump had reached its minimum speed and two-port valves were continuing to close, the pressure relief valve would open to ensure a constant minimum flow through the pump. A BEMS enabled communication of changes in the differential pressure to the secondary pump variable speed drives.

The chillers are simply loaded or offloaded by calculating the prevailing building cooling load, which is achieved by measuring the flow after the secondary pumps and the temperature difference between the secondary chilled water flow and return pipework. This gives

Qc = M x Cp x (Dt)

where Qc = chilled water cooling load (kW)

M = mass flow rate (kg/sec)

Cp = specific heat capacity of water 4.18 (kJ/kg°C)

Dt = temperature of water (°C).

Commissioning

The commissioning exercise was carried out by connecting two electronic flow meters to the BEMS: one located on the main flow pipework from the secondary variable speed pumps, the other on the central bypass.

The differential pressure sensor was initially set to control at the differential pressure predicted by the BSRIA network analysis program. The index circuit valve was opened while all other valves remained closed. At this point, the pressure relief valve was open, allowing the majority of flow to pass through the bypass back to the pump.

By simply deducting the flow rate measured on the bypass flow meter from that measured on the secondary mains flow meter, we could calculate the actual flow rate passing through the coil.

The same exercise was repeated for each coil to ensure that the pressure sensor set point was adequate to deliver the design flow rate under all conditions. We found that the set point valve predicted by the BSRIA program proved to be very accurate.

The commissioning exercise was concluded by opening the first and last valves on the system and checking the overflow and underflow respectively. This was then compared with the results of the network analysis program.

This same exercise can be carried out as part of a preventative maintenance routine.

By carefully analysing system dynamics and accurately sizing two-port valves, the use of regulating valves can be avoided and a more cost effective design solution can be achieved.

Paul Norton is chief mechanical engineer at North Atlantic Engineering Consultants; Chris Parsloe is an independent consultant chrisparsloe@parsloeconsulting.co.uk